报告人：

贺劲松 教授，深圳大学高等研究院

摘  要：

In this talk, we study a new non-linear integrable equation,

which is invariant under scaling of dependent variable and was called the SIdV equation, see Commun. Nonlinear Sci. Numeric. Simulat. 17 (2012) 4155. The order-n kink solution 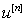 of the SIdV equation, which is associated with the n-soliton solution of the Korteweg-de Vries equation, is constructed by using the n-fold Darboux transformation (DT) from zero “seed” solution. Moreover, we also provide the evolution scenarios of surfaces of revolution associated with the kink-type solutions of the SIdV, where the kink-type solutions play the role of a metric. We put forward two kinds of evolution scenarios for surfaces of revolution associated with two types of kink-type metric (solution) and we study the key properties of these surfaces.

时  间：

12月23日 星期三 9:00—10:30

方  式：

腾讯会议

会议 ID：942 743 554

会议密码：1223

邀请人：

刘青平 教授